Distortion produced by Projection and Rational Equations by Jiyoon Chun

Projection and Rational Functions

is a combination of . We can get these relationships in projective geometry. We will investigated how the rational function relates to projection, and what is the mathematical facts that we can understand and apply in high school mathematics using projective geometry.

What is projection and projective lines?

Think about the railroads. they are parallel in Euclidean geometry. However, when we see the rails, It seems like they meet. More precisely, they meet at the horizon.

Euclidean geometry works fine in many cases, but when we want to draw this, we have to think differently. If we are cling to Euclidean geometry to draw this, then we will ignore the rule of perspective. Before artists followed the rule of perspective, their pictures could not express which one is nearer and which one is farther. In the picture below, the floor looks like vertical.

So, why do we want to deceive ourselves? Although the lines are parallel, they look like meeting at a point on the horizon in the real life. Projective geometry deals with the problem that Euclidean geometry cannot explain (the parallel postulate). Naively saying, projective geometry is assuming that there is an eye which is looking at something. In addition, we want to project the thing what we are watching to another thing such as a line or plane.

Basic Concepts of Projective Lines

Let's go back to the first picture. It seems like two rails meet at a horizon. Then, what does horizon mean? It is at the sight of the eye. Let's see the picture below.

Suppose there is an eye at origin, and we are looking down y=-1. Then,the line passing through origin corresponds to the point of intersection of the line and y=-1. Pay attention to the slopes of the line above. They are getting closer to closer to x-axis. Think about the point on y=-1 which is very far from the origin. The line which connect the point on y=-1 and origin still has an intersection. In other words, the lines through origin always have intersection with y=-1 except when the line is parallel to y=-1.

Intuitively, we can see that y=0 meets y= -1 at horizon. y=0 is at the height of the eye. Therefore, we can say that two parallel lines meet at horizon, which is called point of infinity in projective geometry.

Distortion Produced by Projection

Although there are same sizes of trees are aligned on a railroad, the practical sizes that are observed are different. The nearer, the bigger, the farther, the smaller the trees we see. Thus, distortion happens in projective geometry.

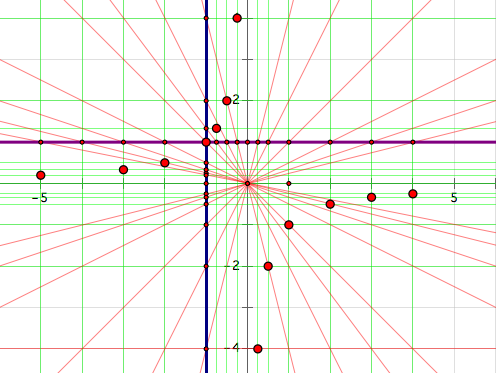
Let's say that the eye is at origin, and want to project y=1 to x=1.

In the picture above, the projected points on x=1 are not equally spaced whereas the points on y=1 are equally spaced. We can see there is distortion while we are projecting points of y=1 onto x=1.

Say the eye can see all 360 degree. Let's mark all the corresponding points on x=1.

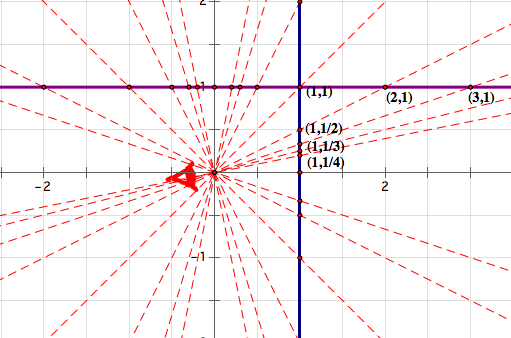
To see how much it is distorted according to the points of y=1, let's plot points. Since we are projecting points of y=1 onto x=1, the coordinates of the new ordered pairs is (x-coordinated of the point of y=1, y-coordinate of the point x=1 which are projected image of the points on y=1). The new ordered pairs would be plotted as the picture below.

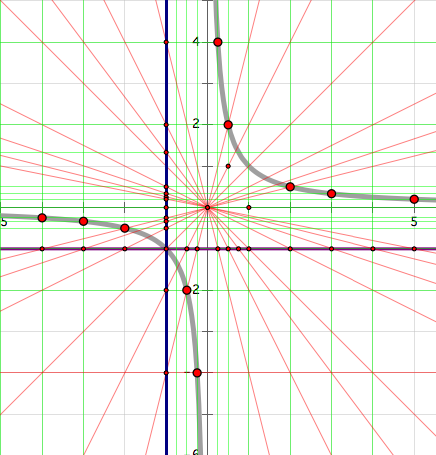
These curves are familiar to us. Yes. It is .

Proof of

Now, we are interested y-coordinate of N' depend on x-coordinate of N. If we make this relation as an ordered pair, we have . This leads to .

For the skeptics, I attach the picture when n<0. The proof is the same.

Asymptotes and the limits of the graph

1) Asymptote y=0

Since y=0 is parallel to y=1, it does not pass through x=1. As we can see in the railroad picture, the point of infinity located at the height of the eye. Suppose that we have a point (n,1) on y=1, and n goes to infinity. Since the point of infinity locates at the height of the eye, the projected points locates at the height of the eye which is on y=0. Thus, we have .

This can also explains why . We assumed that the eye can see 360 degree. When n goes negative infinity, it means that the eye looks back. Although the eye looks back, the height of the eye stays. Therefore, , the height of the eye.

The projection gives a lot of idea of the limits. It is not easy to understand why

and since n goes to the opposite direction. In projective plane, y=0 is actually connected (there is no disconnected part of the line through origin (because the eye can see back and forth)). Thus, although n goes to negative infinity or positive infinity, they are on the same line. This gives an idea why their limits are the same.

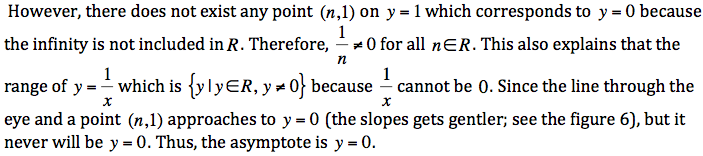
2) Asymptote x=0

When n approaches to 0, we know that the slope of the lines which connect the eye and the points on y=1 gets steeper. However, it cannot give explicit explanation why . It is tricky because is undefined. In projective geometry, it is more clear. The line connecting the eye and (0,1) on y=1 is parallel x=1. It means that we are looking at (0,1) parallel to x=1, which we want to project point on. ntuitively speaking, it is like looking at railroad. Tow parallel lines meet at infinity. Thus, .

Symmetry of the Graph

If we think origin as the eye, then it is easy to explain why is symmetry over origin (the place where the eye is). See the graph below.

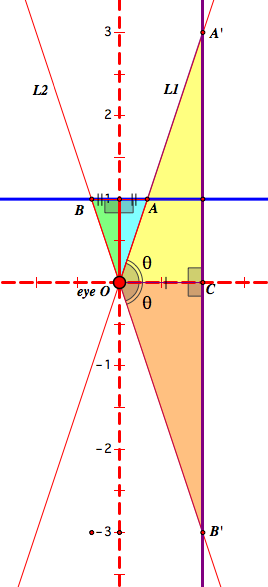
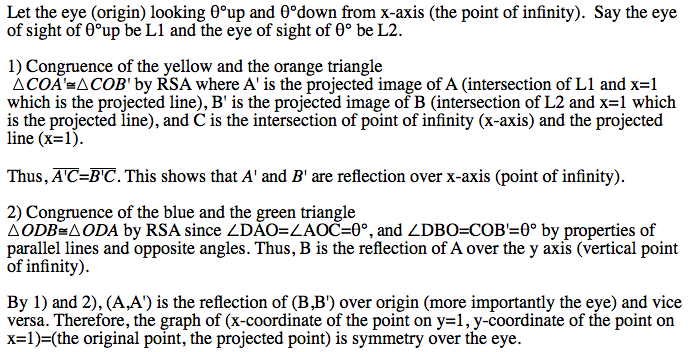
Thus, we can say is symmetry over the eye in the way of projective geometry not just an origin. This gives us an insight of the symmetry of other graphs not just by algebraically.

Locations of the graphs

Now, we understand the place of eye is the key concept of projective line because at the height of the eye, there is an point of infinity. Therefore, origin does not mean a lot in the projective plane. When we refer to quadrants, it will make more sense that the place of eye acts as origin of Cartesian plane.

In the picture above, we can see four quadrants with respect to the place of the eye. Since the eye is at origin, it is hard to see the difference. I will deal with the projection line when the eye is not at origin.

Look at the 2nd and 4th quadrant respect to the eye. On the 4th quadrant, there is not any points to project because we are projecting the points of y=1, and y=1 does not pass the 4th quadrant. Therefore, there are no points plotted. On the 2nd quadrant, the projected line does not exist since we project y=1 to x=1, and x=1 does not cross the 2nd quadrant. Thus, there are no points plotted.

On the 1st quadrant, we see there are two lines: the line we want to project (y=1), and the line on which we want to project the points of y=1 (x=1). Therefore, we know that there would be some points plotted on the 1st quadrant. By the symmetry of the graph, we can see that the graph also is on the 3rd plane.

What if x=-1 instead of x=1?

Let's project x=-1 onto y=1. See the graph below.

It looks like all points lie on . The proof is similar to the previous one. However, I will prove it algebraic way.

Now, we are interested y-coordinate of N' depend on x-coordinate of N. If we make this relation as an ordered pair, we have . This leads to .

How about in case of x=-1, and y=-1? Or x=1, y=-1?

1) When we project point x=-1 to y=-1

By the symmetry of the projective line, we have the same curve with when x=1 and y=1. We also can conclude that if we project points on x=1 to y=-1, we also have the same curve with .

Generalization: When we project the points of x=s onto y=t

Therefore, when we project the point of x=s onto y=t, the set of ordered pairs of the x-coordinate of x=s and the y-coordinate of the projected point onto y=t is .

How do the lines of projection x=s, and y=t explain the shape of ?

Let's compare the two cases when x=1,y=1 and x=4,y=1.

N'=the projected point of Ny=1 onto x=1

N''=the projected point of Ny=1 onto x=4

Since x=4 located on the right side of x=1, the y-coordinate of N'' is bigger that the y-coordinate of N'. Therefore, when we make ordered pairs of the x-coordinate of N and y-coordinate of the projected points, the ordered pairs from N' locates higher than those from N'. Intuitively, we can say the set of ordered pairs locates farther when we project N onto x=4 than x=1 because the line where the images (the projected points) sit on is farther than x=1. See the flash below.

In this way, we can explain the locations of the curves. We know that the curves locates on the 1st and 3rd when st>0, and on the 2nd and 4th quadrant when st<0 where . By the property that we found of the location and symmetry of the curve according to x=s and y=t, we know that we have two curves on the 1st and 3rd quadrant when s>0,t>0 and s<0,t<0. With the same sense, the curve locates on the 2nd and the 4th quadrant when s<0,t>0 and s>0,t<0.

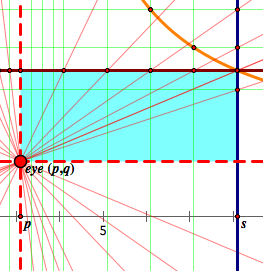
When the eye is not at origin

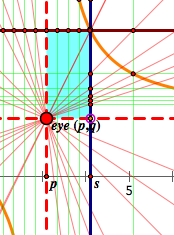
Let's say the eye is at (1,2), and we want to project the points of y=3 onto x=2.

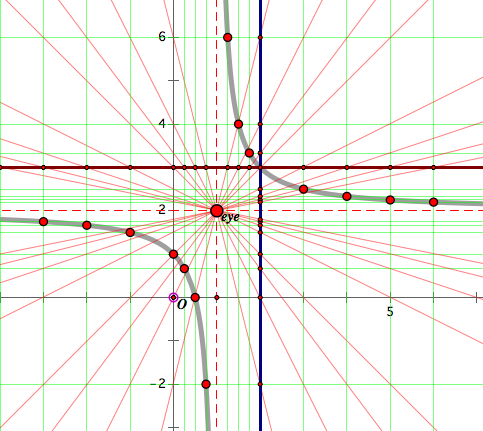
The set of points are .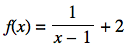

By the property of asymptotes, we know that the intersection of the asymptote is at the place of the eye. Therefore, the asymptotes of the set of the ordered pairs of x-coordinate of a point y=3 and y coordinate of the projected point onto x=2 is x=1 and y=2.

Since the distances of the eye to x=2 and y=3 are the same with , the shape of the graph is the same with .

Generalization: When the eye is at (p,q), and we project the points of y=t onto x=s

1) Asymptotes of the graph: The point of infinity: x=p, y=q (the eye)

The the intersection of the line through the eye and parallel to x=s (which is x=p) project (p,t) to infinity. Therefore, the projected points approaches to x=p vertically.

y=q is parallel to y=t, and passing through the eye. When we think that we are looking at the horizon., the point which is infinitely far from the eye projected on the horizon. Thus, when the x-coordinate goes to infinity, it projected on the horizon., which is the height of the eye, q.

2) Symmetry of the graph

By the property of the congruent triangles, point A and point B are reflection over the eye. Thus, the graph is symmetry over the eye.

3) The equation of the graph

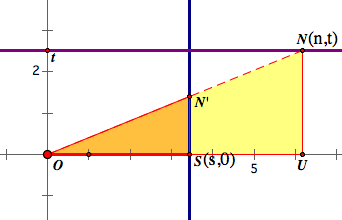
Let's find y-coordinate of k which is the projection point of ny=t onto x=t.

Since the yellow and the orange triangles are similar, the slopes of the hypotenuses of the two triangles are the same.

The slope of the hypotenuse of the yellow triangle=the slope of the hypotenuse of the orange triangle. This gives

Therefore, (x-coordinate of (n,t), y-coordinate of (s,k)=(n,k)=.

This gives us that the equation of the set of ordered pair (n,k) is .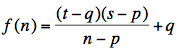

If we rewrite this as an x-y relationship, this gives the familiar equation such that .

4) The location of the graph

As I mentioned above, when (t-q)(s-p)>0, the curves locate on the 1st and the 3rd quadrant respect to the eye. If (t-q)(s-p)<0, the curves are on the 2nd and 4th quadrant.

5) The shape of the graph

The bigger |(t-q)(s-p)|is, the farther the curves are from the eye. |(t-q)(s-p)| is the area of the rectangle of the base (s-p) and the height (t-q).

Compare the two pictures above. When the blue rectangle of the area |(t-q)(s-p)|, the projected points on x=s is spread out. When the blue rectangle is small, the projected points on x=s are squeezed in for equally spaced points on y=t. See the flash below.

Let's fix x=s and move y=t as in the flash above. We can see the slope of the eyesight is getting gentler as it approaches to y=q. For the same spaced points, there will be more projected points when |t-q| gets smaller. Therefore, the ordered pairs of (n, k)= would be squeezed in. Although this is not a formal proof, this gives us nice intuitive idea of the shape according to |(t-q)(s-p)|.

One more interest thing is the case when the lines approach to the eye. The curves disappear because all the points were sent to the point of infinity. The flash above gives us nice visual explanation about the asymptotes If you want to play this on GSP file, click here.

6) The graph movement: Moving the eye for fixed distance between the eye and the two lines ( which means (t-q) and (s-p) are fixed)

Since |(t-q)(s-p)| affects the shape of the graph, the shape of the curves stay as long as the distance between the eye and x=s, y=t are fixed.

Comparison of and projection of y=t onto x=s at the eye (p,q)

The way of understanding algebraically and projective geometrically are little bit different. See the table below.

		projection of y=t onto x=s at the eye (p,q)
Equation form
Domain
reason	The denominator cannot be zero.	When we connect the eye and (p,n) on y=t, the line is parallel to x=s. Therefore, (p,n) cannot be projected on the line x=s. The line connecting of the eye and (p,n) and x=s meets at infinity. Since infinity does not exist in R, the function is undefined when x=p.
Range
reason	Since cannot be 0, y cannot be .	y=q is parallel to y=t. In projective geometry, parallel lines meet at the height of the eye, the horizon. Therefore, the line through the eye and (n,t) on y=t meet y=q when n goes infinity. Since there is no infinity in R, y=q cannot hit any point on y=q.
Asymptotes	,	,
reason	Since the denominator cannot be zero, there would no y-value corresponding . is an asymptote because .	(p,t) corresponds to the infinity since the eye is looking at the point vertically up and parallel to the projected line. (n,t) when (n,t) projected to the horizon, at the height of the eye, y=q
Symmetry	Over	Over (p,q)
reason		By the congruency of the triangles
Location	* the 1st and the 3rd quadrant when >0 respect to * the 2nd and the 4th quadrant when <0 respect to	* the 1st and 3rd quadrant when (t-q)(s-p)>0 respect to the eye * the 2nd and 4th quadrant when (t-q)(s-p)<0 respect to the eye
reason	Thus, if there is a curve on the 1st quadrant, there must be the symmetry curve on the 3rd quadrant by the property of the odd functions.	When the rectangle of the base (s-p) and the height (t-q) are on the 1st quadrant respect to the eye, the projected points are on the rectangle. Therefore, the ordered pairs are on the 1st quadrant. This counts for every quadrant.
Shape	The bigger is, the farther the curves from .	The bigger \|(t-q)(s-p)\|is, the farther the curves are from the eye.
reason	Since is multiplied to , f(x) is also multiplied by .	When the blue rectangle of the area \|(t-q)(s-p)\|, the projected points on x=s is spread out.
Graph movement horizontally k and vertically m	Substitute (x-k) for x, and (y-m) for y	Move the eye (p,q) horizontally k, vertically m with preserving the distance between the eye and the lines.

As we see in the table above, understanding rational function projective geometrically makes more sense than algebraic way.

Personal opinion

I first learned projective geometry this year, and I was fascinated because I could find the other way to understand the limits. Although I could solve limit problems, it is hard to understand the concept of limits. I remember I just accepted what teachers said, and I also repeated the same thing to my own students.

Students learn projective geometry very early in art class. When they draw or paint the picture, they first figure out where the eye is looking at the place that they want to put on the canvas. In addition, they also know where the horizon., and the point of infinity. They already experience the perspective view. Thus, projective geometry is not totally new thing to the students. Rather, it is more familiar than Euclidean geometry in the sense that we always see things in a perspective view.

Understanding rational functions in projective geometry way enable us to supply visual reasons about the asymptotes and the concepts of infinity. Some might think projective geometry could be to hard to the students simply because they did not learn it in high school or simply because it is not in the curriculum. When I taught rational functions to the students, I knew that there are so many students who are embarrassed by the strange looking curves. If we teach projective geometry and help them understand rational functions in this way, I think students can understand better since projective geometry is on the extension of common sense.

Reference

The four pillars geometry by John Stillwel

RETURN